O-Ring Analysis Using Advanced Elastomeric Material Laws with LS-DYNA

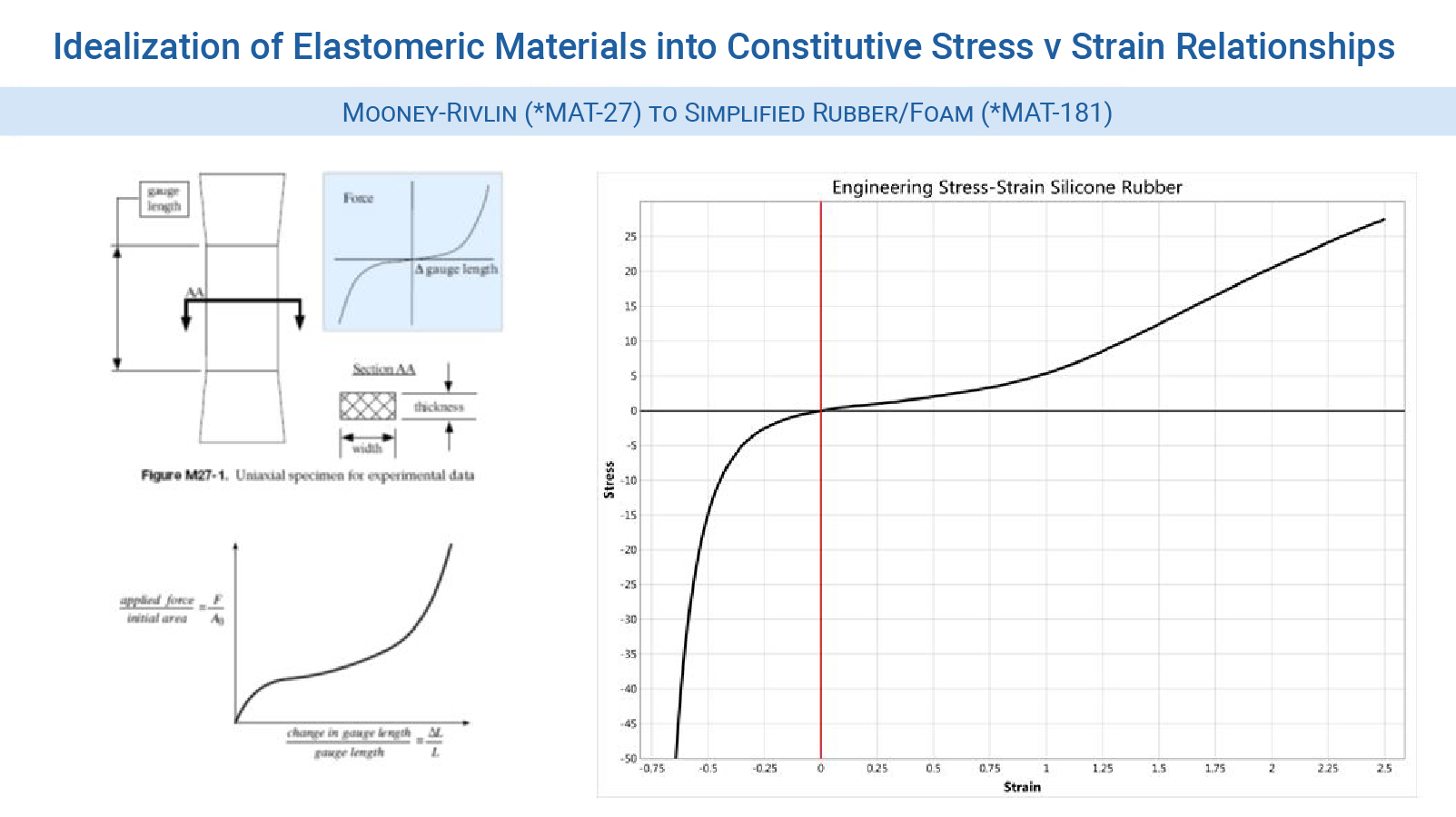
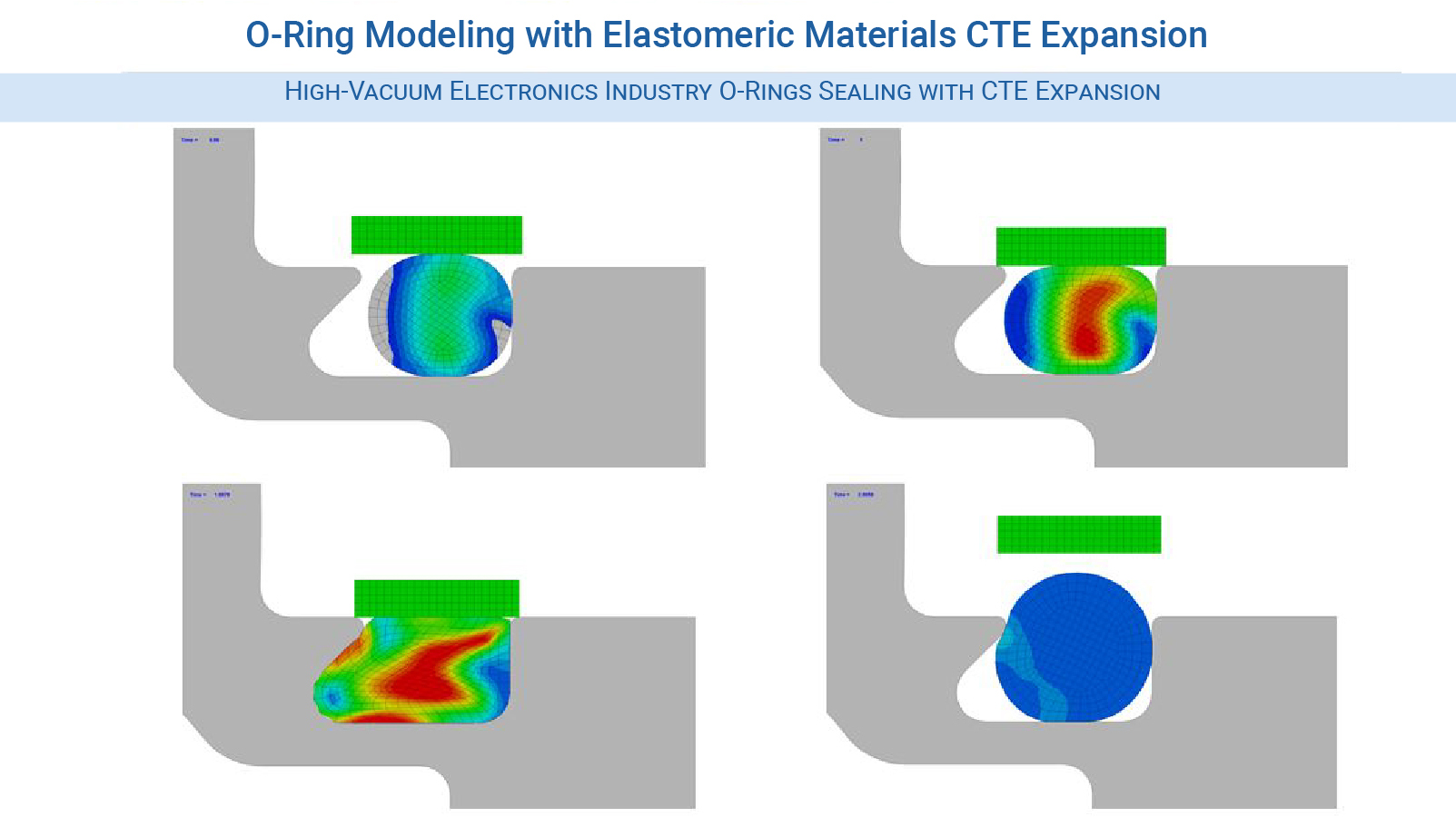
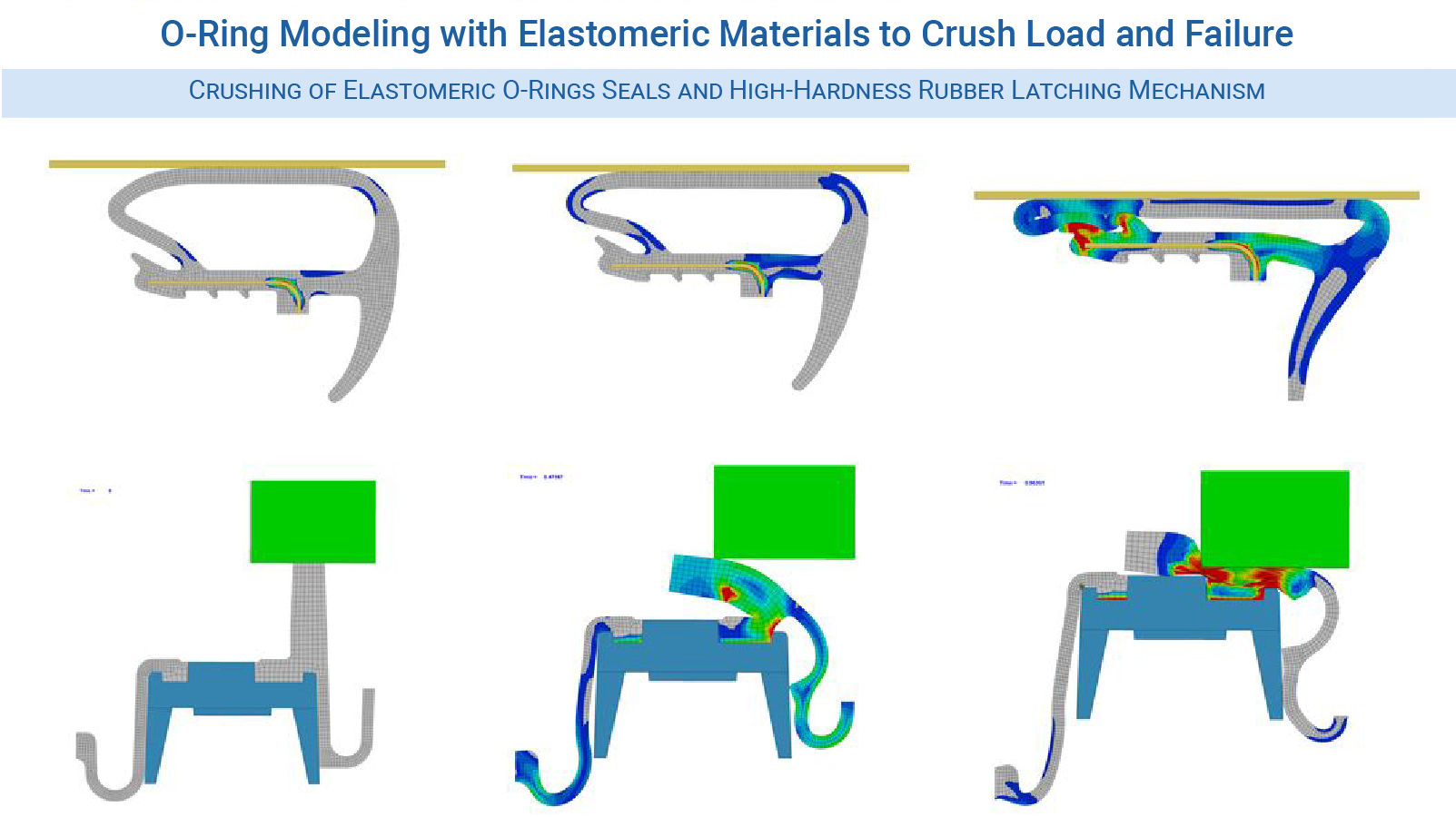
The analysis of O-rings has historically been difficult since the modeling process involves large displacement and large nonlinear strains couple to multi-body contact between the O-ring, its gland cavity and the mating part. In our prior engineering services work, we would have to run the model in explicit mode and just live with the long run times even through the model was axisymmetric. In the last couple of years, the implicit capabilities of LS-DYNA have significantly grown and we can now run such models much faster and with no inertia effects.

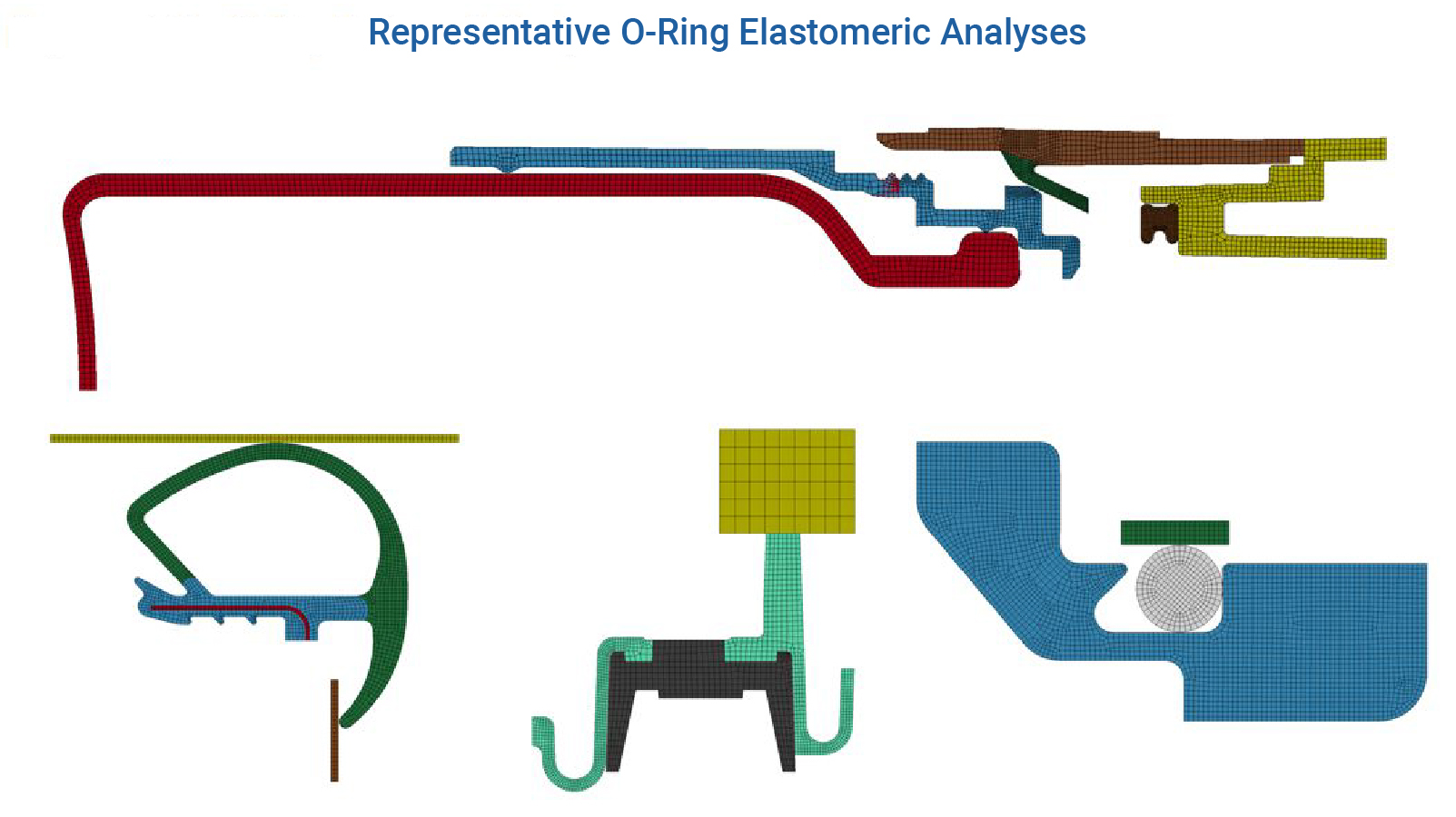
In this case study note, we’ll cover four different O-ring simulations that we have done as LS-DYNA consulting services projects. Each project presents a different type of challenge using different material models and element types from 2-D plane strain to axisymmetric. Figure 1 provides a snapshot of the four models discussed in this note.