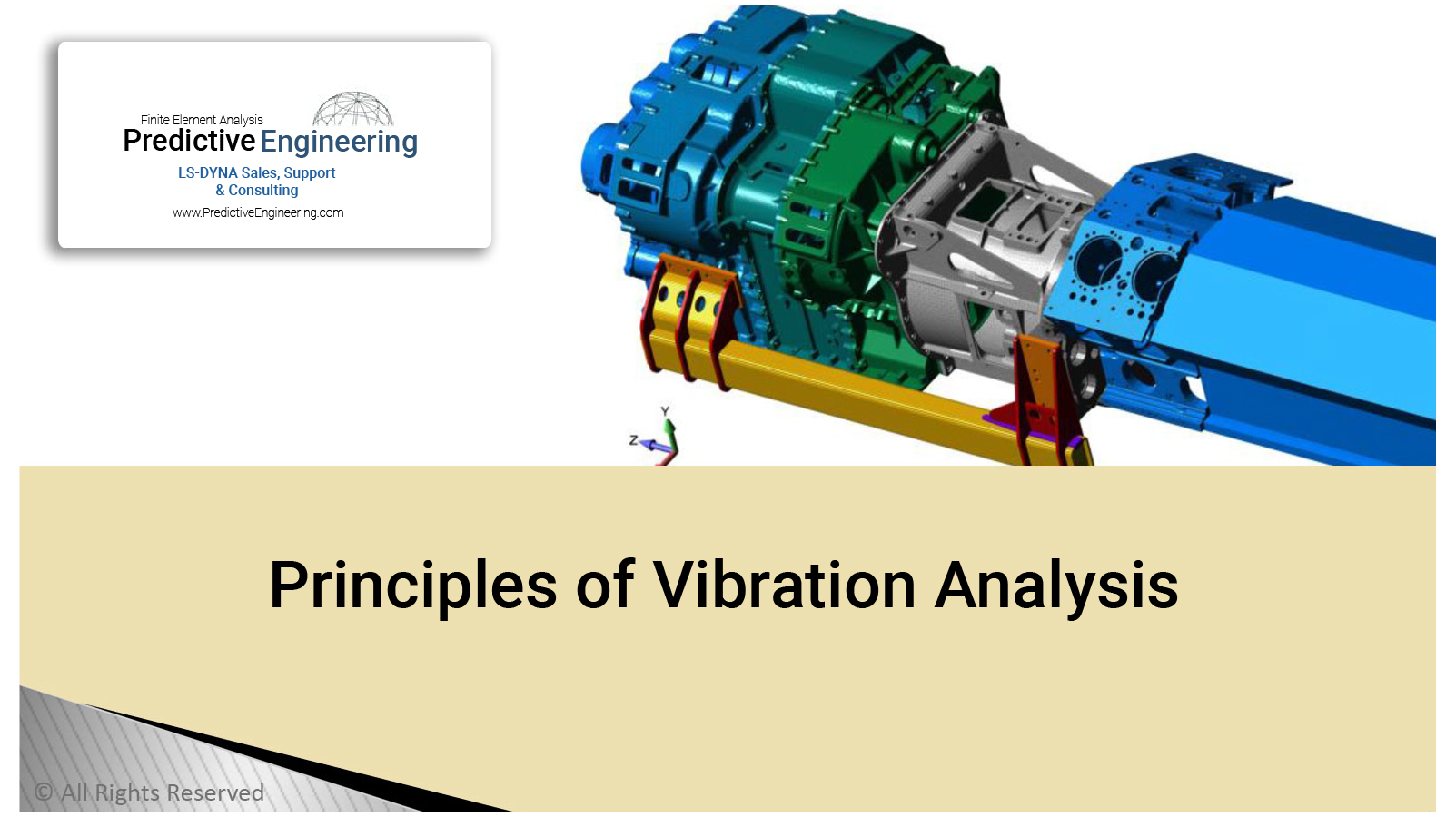
Vibration analysis is a huge topic and is easily the second most common type of FEA analysis after the basic static stress analysis. Within the field of vibration analysis, the most common type of analysis is that based on the linear behavior of the structure or system during its operation. That is, its stress/strain response is linear and when a load is removed, the structure returns to its original position in a stress/strain free condition.
What’s Covered: Foundation of Frequency Analysis, Standard Normal Modes Analysis, Modal Frequency Analysis, PSD Analysis, Direct Transient Analysis, Model Checkout (mass and constraints) and Additional Reading
An Excerpt From the White Paper: Standard Normal Modes Analysis: To see how this is applied in practice, we will run through an analysis project from start to finish (Normal Modes, Modal Frequency, PSD and Direct Transient). The model has been tweaked to protect the innocent.